V11.] DISCUSSION OF THE DATA OF STATURE. 127
four independent values of b, which are respectively
1.01, 1.01, 1.20, and 1.08 ; the mean of these is 1.07.
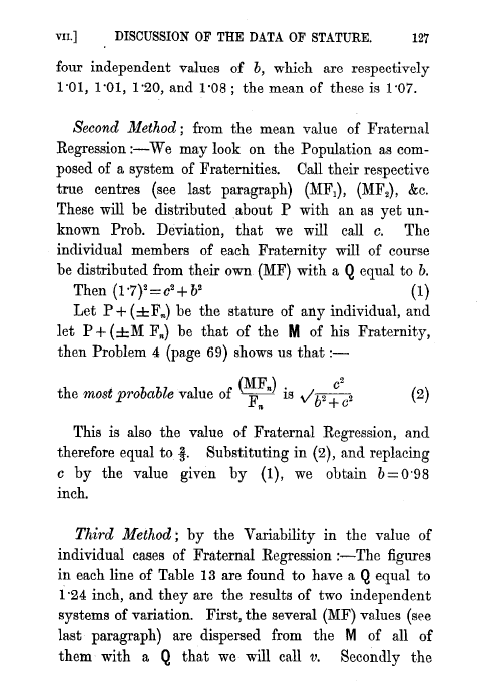
Second Method ; from the mean value of Fraternal Regression:-We may look on the Population as composed of a system of Fraternities. Call their respective true centres (see last paragraph) (MF,), (MF2), &c. These will be distributed about P with an as yet unknown Prob. Deviation, that we will call c. The individual members of each Fraternity will of course be distributed from their own (MF) with a Q equal to b.
Then (1.7)2=c2+b2 (1)
Let P + (±F,,,) be the stature of any individual, and let P + (±M Fn) be that of the M of his Fraternity, then Problem 4 (page 69) shows us that :
This is also the value of Fraternal Regression, and therefore equal to ~. Substituting in (2), and replacing c by the value given by (1), we obtain b=0'98 inch.
Third Method ; by the Variability in the value of individual cases of Fraternal Regression :-The figures in each line of Table 13 are found to have a Q equal to 1.24 inch, and they are the results of two independent systems of variation. First, the several (MF) values (see last paragraph) are dispersed from the M of all of them with a Q that we will call v. Secondly the
the most probable value F
of (CIF") is 2 c2
„ b + c2
(2)