| ||||||
OCR Rendition - approximate
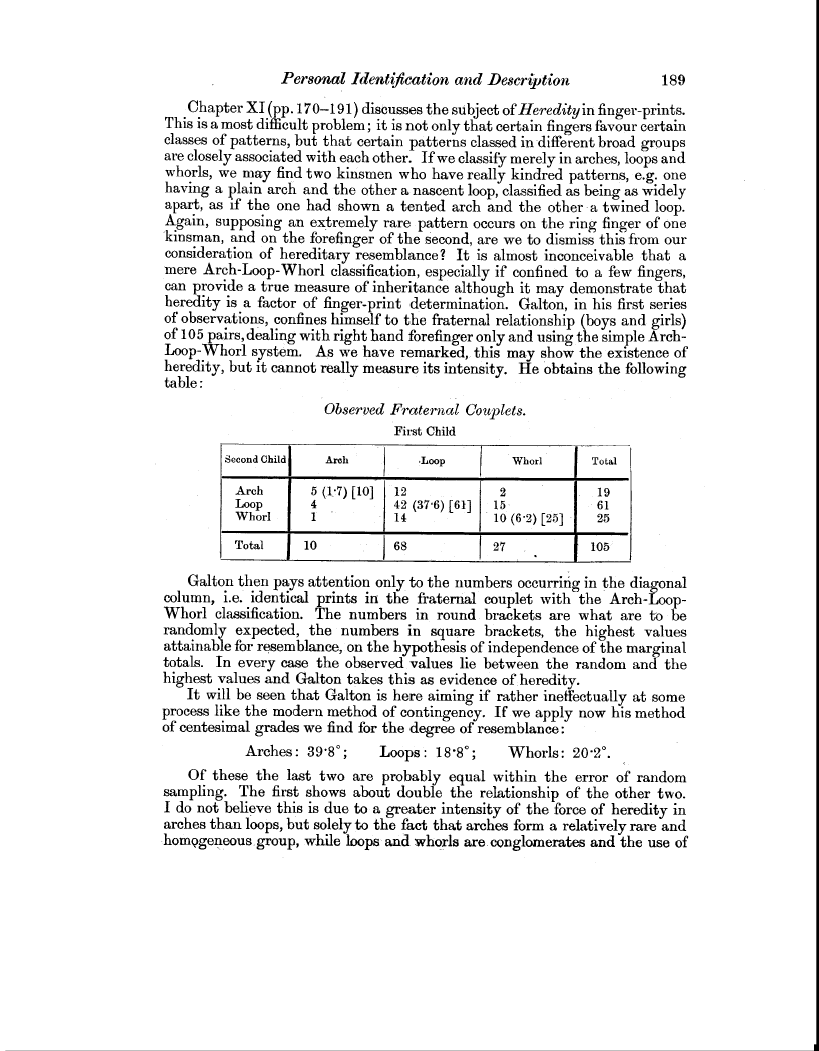
Personal Identification and Description 189 Chapter XI (pp. 170-191) discusses the subject of Heredity in finger-prints. This is a most difficult problem; it is not only that certain fingers favour certain classes of patterns, but that certain patterns classed in different broad groups are closely associated with each other. If we classify merely in arches, loops and whorls, we may find two kinsmen who have really kindred patterns, e.g. one having a plain arch and the other a nascent loop, classified as being as widely apart, as if the one had shown a tented arch and the other -a twined loop. Again, supposing an extremely rare pattern occurs on the ring finger of one kinsman, and on the forefinger of the second, are we to dismiss this from our consideration of hereditary resemblance? It is almost inconceivable that a mere Arch-Loop-Whorl classification, especially if confined to a few fingers, can provide a true measure of inheritance although it may demonstrate that heredity is a factor of finger-print determination. Galton, in his first series of observations, confines himself to the fraternal relationship (boys and girls) of 105 pairs, dealing with right hand forefinger only and using the simple ArchLoop-Whorl system. As we have remarked, this may show the existence of heredity, but it cannot really measure its intensity. He obtains the following table Observed Fraternal Couplets. First Child
Galton then pays attention only to the numbers occurring in the diagonal column, i.e. identical prints in the fraternal couplet with the Arch-LoopWhorl classification. The numbers in round brackets are what are to be randomly expected, the numbers in square brackets, the highest values attainable for resemblance, on the hypothesis of independence of the marginal totals. In every case the observed values lie between the random and the highest values and Galton takes this as evidence of heredity. It will be seen that Galton is here aiming if rather ineffectually at some process like the modern method of contingency. If we apply now his method of centesimal grades we find for the degree of resemblance: Arches : 39-8'; Loops: 18'8° ; Whorls : 20.2°. Of these the last two are probably equal within the error of random sampling. The first shows about double the relationship of the other two. I do not believe this is due to a greater intensity of the force of heredity in arches than loops, but solely to the fact that arches form a relatively rare and hom9geneous.group, while loops and whorls aree conglomerates and the use of
| |||||||||||||||||||||||||||||||