| ||||||
OCR Rendition - approximate
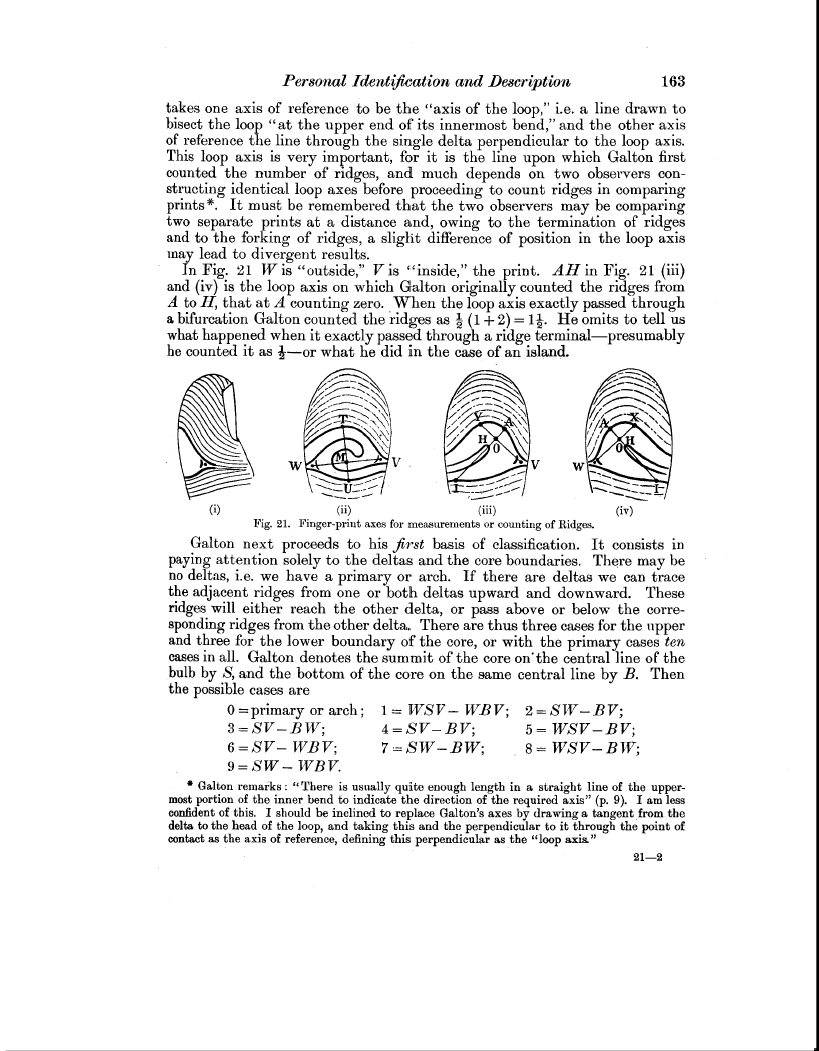
Personal Identification and Description 163 takes one axis of reference to be the "axis of the loop," i.e. a line drawn to bisect the loop "at the upper end of its innermost bend," and the other axis of reference the line through the single delta perpendicular to the loop axis. This loop axis is very important, for it is the line upon which Galton first counted the number of ridges, and much depends on two observers constructing identical loop axes before proceeding to count ridges in comparing prints*. It must be remembered that the two observers may be comparing two separate prints at a distance and, owing to the termination of ridges and to the forking of ridges, a slight difference of position in the loop axis may lead to divergent results. In Fig. 21 W is "outside," V is "inside," the print. AH in Fig. 21 (iii) and (iv) is the loop axis on which Galton originally counted the ridges from A to H, that at A counting zero. When the loop axis exactly passed through a bifurcation Galton counted the ridges as 2 (1 + 2) =12. He omits to tell us what happened when it exactly passed through a ridge terminal-presumably he counted it as I--or what he did in the case of an island. (i) (ii) (iii) Fig. 21. Finger-print axes for measurements or counting of Ridges. Galton next proceeds to his first basis of classification. It consists in paying attention solely to the deltas and the core boundaries. There may be no deltas, i.e. we have a primary or arch. If there are deltas we can trace the adjacent ridges from one or both deltas upward and downward. These ridges will either reach the other delta, or pass above or below the corresponding ridges from the other delta. There are thus three cases for the upper and three for the lower boundary of the core, or with the primary cases ten cases in all. Galton denotes the summit of the core on'the central line of the bulb by S, and the bottom of the core on the same central line by B. Then the possible cases are 0 =primary or arch; 1= WSV - WBV; 2 = SW-BV; 3=SV-BW; 4=SV-BV; 5=WSV-BV; 6=SV-WBV; 7=SW-BW; 8=WSV-BW; 9=SW- WBV. Galton remarks : "There is usually quite enough length in a straight line of the uppermost portion of the inner bend to indicate the direction of the required axis" (p. 9). I am less confident of this. I should be inclined to replace Galton's axes by drawing a tangentt from the delta to the head of the loop, and taking this and the perpendicular to it through the point of contact as the axis of reference, defining this perpendicular as the "loop axis."     21-2
|