| ||||||
OCR Rendition - approximate
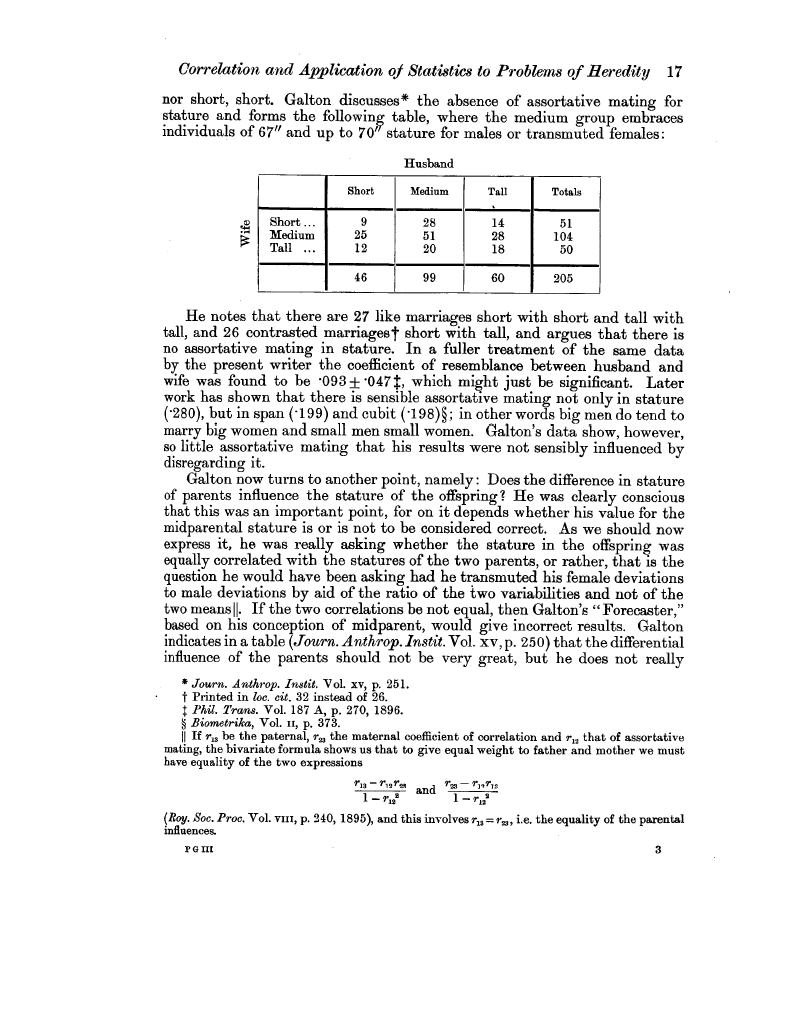
Correlation and Application of Statistics to Problems of Beredity 17 nor short, short. Galton discusses* the absence of assortative mating for stature and forms the followin table, where the medium group embraces individuals of 67" and up to 70' stature for males or transmuted females: Husband
He notes that there are 27 like marriages short with short and tall with tall, and 26 contrasted marriages' short with tall, and argues that there is no assortative mating in stature. In a fuller treatment of the same data by the present writer the coefficient of resemblance between husband and wife was found to be •093 +,047 $, which might just be significant. Later work has shown that there is sensible assortative mating not only in stature (280), but in span (199) and cubit (198)§; in other words big men do tend to marry big women and small men small women. Galton's data show, however, so little assortative mating that his results were not sensibly influenced by disregarding it. Galton now turns to another point, namely : Does the difference in stature of parents influence the stature of the offspring? He was clearly conscious that this was an important point, for on it depends whether his value for the midparental stature is or is not to be considered correct. As we should now express it, he was really asking whether the stature in the offspring was equally correlated with the statures of the two parents, or rather, that is the question he would have been asking had he transmuted his female deviations to male deviations by aid of the ratio of the two variabilities and not of the two means II. If the two correlations be not equal, then Galton's "Forecaster," based on his conception of midparent, would give incorrect results. Galton indicates in a table (Journ. Anthrop. Instit. Vol. xv, p. 250) that the differential influence of the parents should not be very great, but he does not really * Journ. Anthrop. Instit. Vol. xv, p. 251. t Printed in loc. cit. 32 instead of 26. Phil. Trans. Vol. 187 A, p. 270, 1896. Biometrika, Vol. Ii, p. 373. II If r13 be the paternal, ru the maternal coefficient of correlation and r1, that of assortative mating, the bivariate formula shows us that to give equal weight to father and mother we must have equality of the two expressions r1, - r12r2R and r23 1 - r122 1 - r122 (Roy. Soc. Proc. Vol. vin, p. 240, 1895), and this involves rl,-r2 , i.e. the equality of the parental influences. P G3 III 3
|