300 Life and Letters of Francis Galton
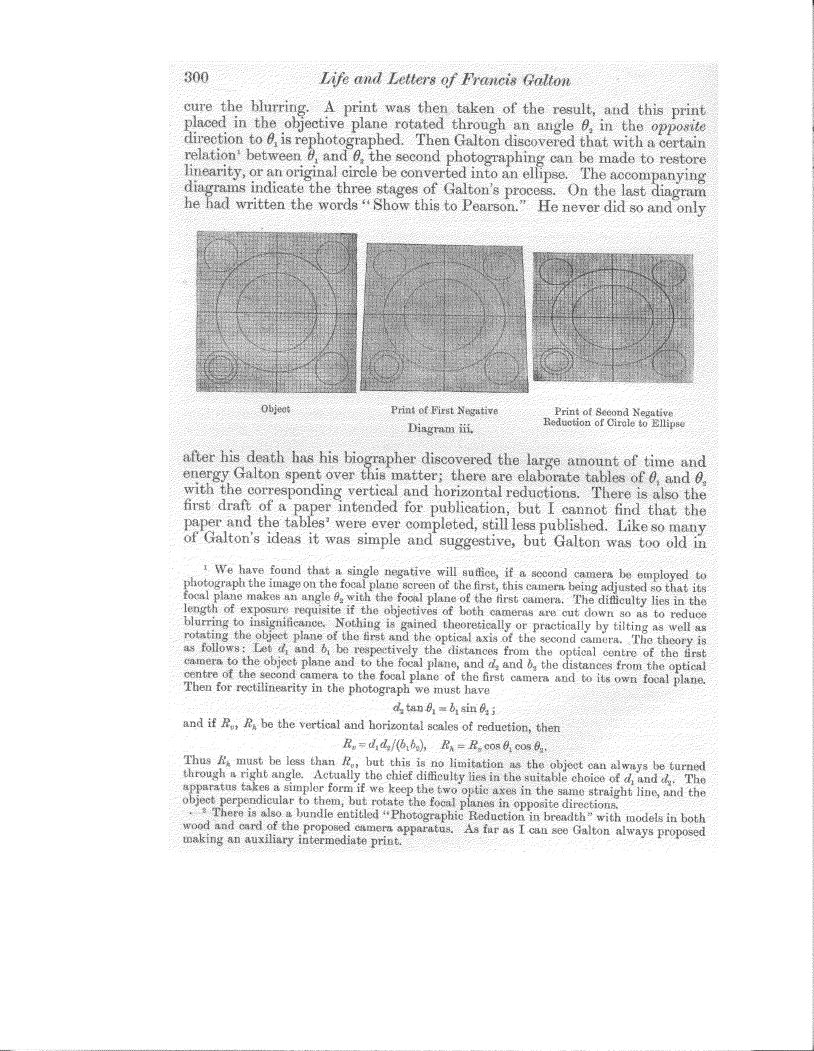
cure the blurring. A print was then taken of the result, and this print placed in the objective plane rotated through an angle 0, in the opposite direction to 0, is rephotographed. Then Galton discovered that with a certain relation' between 0, and 0, the second photographing can be made to restore linearity, or an original circle be converted into an ellipse. The accompanying diagrams indicate the three stages of Galton's process. On the last diagram he had written the words " Show this to Pearson." He never did so and only
after his death has his biographer discovered the large amount of time and energy Galton spent over this matter; there are elaborate tables of 0, and 0., with the corresponding vertical and horizontal reductions. There is also the first draft of a paper intended for publication, but I cannot find that the paper and the tables' were ever completed, still less published. Like so many of Galton's ideas it was simple and suggestive, but Galton was too old in
' We have found that a single negative will suffice, if a second camera be employed to photograph the image on the focal plane screen of the first, this camera being adjusted so that its focal plane makes an angle 0, with the focal plane of the first camera. The difficulty lies in the length of exposure requisite if the objectives of both cameras are cut down so as to reduce blurring to insignificance. Nothing is gained theoretically or practically by tilting as well as rotating the object plane of the first and the optical axis of the second camera. _The theory is as follows : Let d, and b, be respectively the distances from the optical centre of the first camera to the object plane and to the focal plane, and d, and b, the distances from the optical centre of the second camera to the focal plane of the first camera and to its own focal plane. Then for rectilinearity in the photograph we must have
d,tan9,=b,sin02;
and if R,,, Rh be the vertical and horizontal scales of reduction, then R„ = d, d,/(b, b,), Rh = R, cos 0, cos 0,.
Thus Rh must be less than R,,, but this is no limitation as the object can always be turned through a right angle. Actually the chief difficulty lies in the suitable choice of d, and d,. The apparatus takes a simpler form if we keep the two optic axes in the same straight line, and the object perpendicular to them, but rotate the focal planes in opposite directions.
. 2 There is also a bundle entitled "Photographic Reduction in breadth" with models in both wood and card of the proposed camera apparatus. As far as I can see Galton always proposed making an auxiliary intermediate print.
object
Print of First Negative
Diagram iii.
Print of Second Negative
Reduction of Circle to Ellipse